December 23, 2024
5 min read
A Little Math Can Streamline Holiday Cookie Making
Making cookies is time- and labor-intensive. Here’s how a little math can make it easier and less wasteful this holiday season

A cookie cutter that creates a tessellation can save time and use up more dough.
When our children were little, we spent a lot of time in the kitchen together. We used the occasions to surreptitiously teach them about science: heat and temperature, fluid flow, density, viscosity, physical changes, chemical reactions, acids and bases. We told them how these properties combined to magically make cakes rise with carbon dioxide; we told them about yeasts and other interesting microorganisms.
We also talked about math: weights and measures, areas and volumes. Pizza is a great way to introduce fractions. We enjoyed baking cookies together and used many different cookie cutters. Our son loved dinosaurs so we had dinosaur cookie cutters, but also flowers, hearts, stars and animals. An interesting conundrum came up when we used the cookie cutters: they left gaps between each shape we cut out. To use up all the dough, it had to be rerolled. But then it warmed up and had to be chilled before we could cut it again. This took time.
There had to be a more efficient way to divide the dough.
On supporting science journalism
If you’re enjoying this article, consider supporting our award-winning journalism by subscribing. By purchasing a subscription you are helping to ensure the future of impactful stories about the discoveries and ideas shaping our world today.
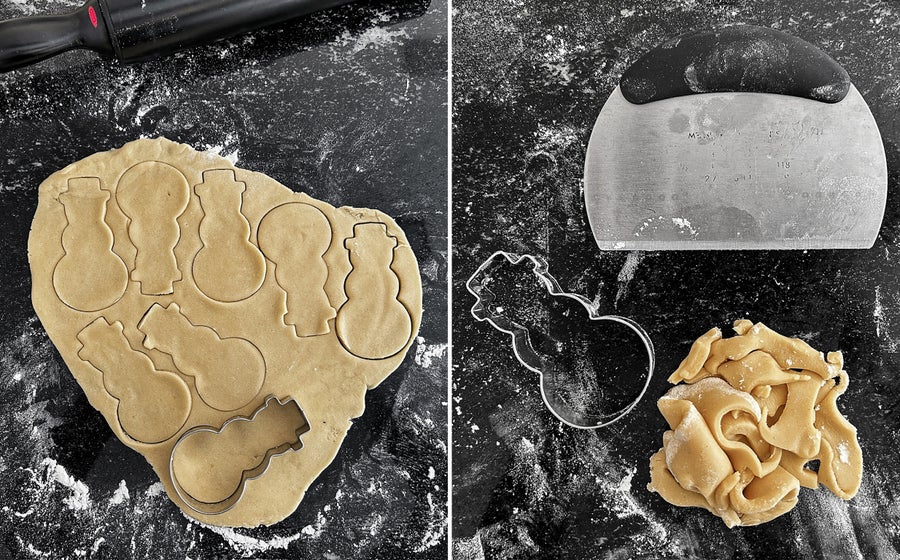
Making cookies using more traditional cookie cutters often leaves a lot of leftover dough.
Being a mathematician, I had heard about tilings and tessellations, which refer to the covering of a surface, using one or more geometric shapes, with no overlaps and no gaps. That was the answer to the cookie cutter problem!
So I looked for tessellating cookie cutters but found only squares and hexagons. They work but are not particularly interesting, as far as shapes go. And then, about 15 years ago, the first 3D printers arrived. It was possible to make custom cookie cutters, and then it became a matter of choosing the shape. Many figures can be used to tessellate, such as regular or irregular triangles, rectangles or some irregular pentagons. Some of the irregular pentagons were discovered by the late amateur mathematician Marjorie Rice and can be seen in Mathemalchemy, an artistic and mathematical exhibit with collaborators including Duke University mathematician Ingrid Daubechies and textile artist Dominique Ehrmann. The exhibit also shows a tessellating cookie cutter using the symbol for pi, suitably tweaked by Daubechies.
There are other examples; Islamic art includes beautiful and creative designs using pentagonal symmetry.
If we join two or more figures then there are many more options. For example, octagons and squares, or pentagons and rhombuses, or all the amazing work by M.C. Escher. But not all make for good cookies; we made a cookie cutter in the shape of one of Escher’s lizards but the legs broke when we separated the figures.
All these tessellations are periodic, which means they have a repeating pattern. In the 1970s Roger Penrose found two figures called kites and darts that tile a plane only aperiodically (meaning that all possible configurations are nonperiodic) if some local rules, specifying which sides of the dart can touch each other, are obeyed. But if the rules are not obeyed then they tile periodically. And this is perfect for a cookie cutter because the raw dough is tiled periodically, and then the cookies can be placed periodically or nonperiodically. There is more information about Penrose tiles in the video channel Veritasium and at a Web page titled “A Penrose-type Islamic Interlacing Pattern.”

A tessellating cookie cutter, such as this one in the shape of a kite and dart, creates a nice pattern and uses up more dough than other shapes.
A couple of years ago, using a 3D printer, with a lot of help from my friends, I made a cookie cutter in the shape of a kite and dart together in a way that tiles periodically. After baking, the pieces can be placed periodically or nonperiodically. This worked beautifully.
And then the Hat arrived.
For about 50 years mathematicians wondered if there could be one single figure that tiled a plane aperiodically. And in 2023 David Smith, Craig Kaplan, Chaim Goodman-Strauss and Samuel Myers managed to find the elusive aperiodic monotile, which they called “the Hat” or “einstein.”
My first impulse was to make a cookie cutter in the shape of the Hat. It turned out to be extremely difficult to place the pieces without gaps or overlaps. But the people who found the Hat actually also realized it could be transformed into an infinite number of aperiodic monotiles, as reported by Craig Kaplan. Three of the monotiles can tile both periodically and nonperiodically, and they are perfect as cookie cutters.
A chevron that Smith and crew called “Tile (0,1)” is easy to make, easy to use on the dough, and the baked cookies are easy to place in beautiful patterns, especially if they are decorated with different colors. Another perfect candidate is what the authors called “Tile (1,1).” This figure tiles periodically when combined with its mirror image but only aperiodically if the mirror image is not allowed. Therefore, the perfect cookie cutter has two of these figures, one normal and one reflected.
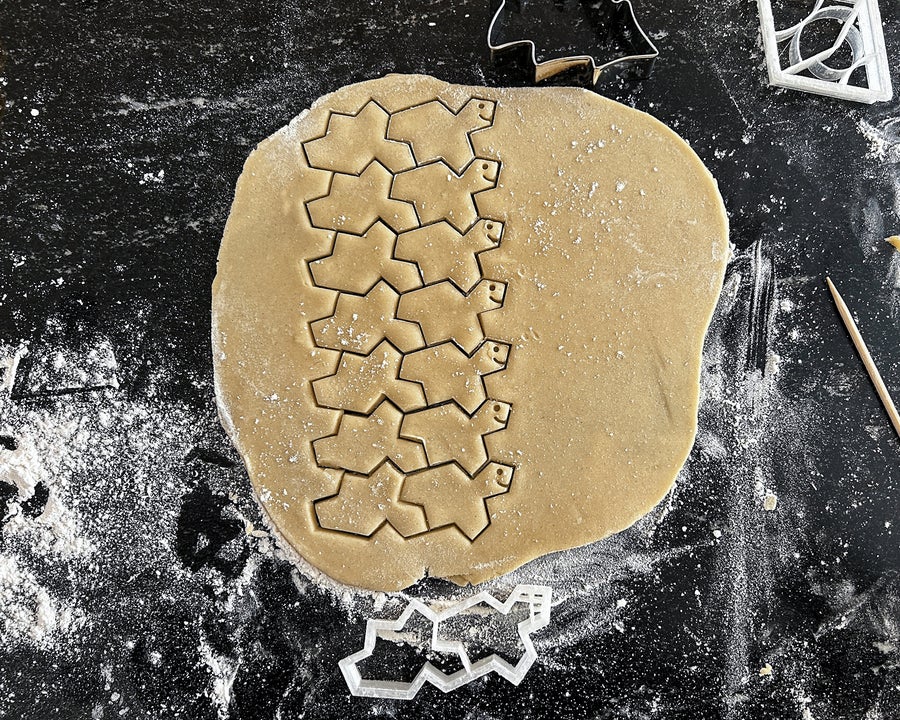
A cookie cutter based on the Hat, discovered by David Smith, makes a tessellating pattern that wastes little dough.
Smith and collaborators also invented a category of curved aperiodic monotiles, which they called “Spectres,” which could also be turned into cookie cutters, as could the recently discovered soft cells. In each of these instances, if you are careful, you can cut your cookie dough with minimal rerolling and waste.
As you cook and bake over the holidays, if you can find a tessellating cookie cutter and if you involve your children, you can take the time you would have spent rerolling dough to tell them about science and math. In our case, our children are grown, but my collection of tessellating cookie cutters and I are excited to share the wonders of math and science when our grandchildren arrive.
This is an opinion and analysis article, and the views expressed by the author or authors are not necessarily those of Scientific American.